Diffraction is the bending of light when light encounters an obstacle of size which is in the range of wavelength of light. Hoping that you are clear with the basic idea of diffraction, lets move ahead. Based on the natural limits of diffraction. Expressions describing the vortex beams that are generated by the process of Fresnel diffraction of a Gaussian beam incident out of waist on fork-shaped gratings of arbitrary integer charge p, and vortex spots in the case of Fraunhofer diffraction by these gratings, are deduced. When calculating diffraction patterns, we further distinguish between near-field (Fresnel) and far-field (Fraunhofer) diffraction. In the far-field approximation, the source and observation screen are sufficiently far away from the diffracting aperture that we can treat the wavefronts as plane.
In classical physics, we can classify optical phenomena into one of two categories: ray optics and wave optics.
Light is a transverse electromagnetic wave. In many situations, the wavelengths of the light being studied are very small compared to the dimensions of the equipment used to study the light. Under these conditions we can make an approximation called geometrical optics or ray optics. Consider the wavelength scale of light waves. Wavelengths in the middle of the visible band are on the order of 500 nm. So a laser beam with a diameter of 1 mm has a diameter of 2000 wavelengths. Individual atoms in a solid are separated by distance on the order of 0.1 nm. So, as far as visible light is concerned, matter is quasi-continuous.
If the wavelengths of the light become comparable to the dimensions of the equipment, then we study optical phenomena using the classical theory of radiation, or wave optics. Wave optics contains all of ray optics, but the mathematical treatment is much more involved.
Diffraction and interference are phenomena observed with all waves. Diffraction can only be observed with waves traveling in two or three dimensions. (We already encountered interference when studying mechanical waves on a string and sound waves in physics 221.)
Diffraction is the tendency of a wave emitted from a finite source or passing through a finite aperture to spread out as it propagates. Diffraction results from the interference of an infinite number of waves emitted by a continuous distribution of source points in two or three dimensions. Huygens' principle lets us treat wave propagation by considering every point on a wave front to be a secondary source of spherical wavelets. These waveletspropagate outward with the characteristic speed of the wave. The wavelets emitted by all points on the wave front interfere with each other to produce the traveling wave. Huygens' principle also holds for electromagnetic waves. When studying the propagation of light, we can replace any wave front by a collection of sources distributed uniformly over the wave front, radiating in phase.
When light passes through a small opening, comparable in size to the wavelength λ of the light, in an otherwise opaque obstacle, the wave front on the other side of the opening resembles the wave front shown on the right.
The light spreads around the edges of the obstacle. This is the phenomenon of diffraction. Diffraction is a wave phenomenon and is also observed with water waves in a ripple tank.
Water waves in a ripple tank
| A single large slit | A single small slit |
Please watch: Apertures and Diffraction - Exploring Wave Motion (YouTube)
The single slit
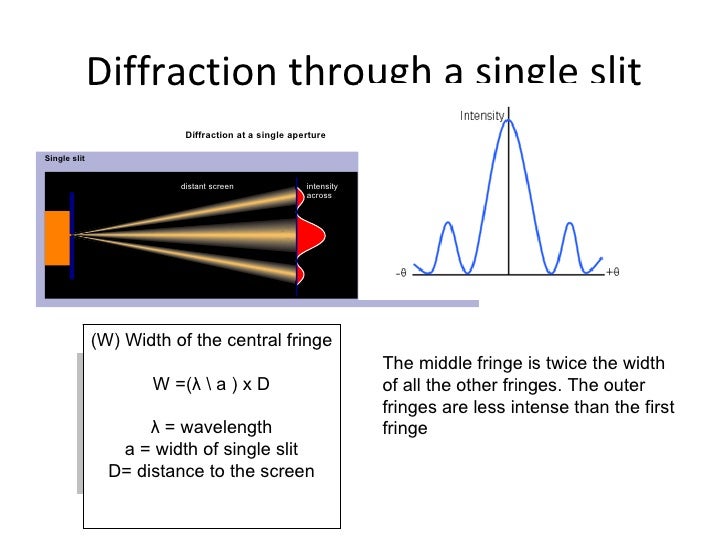
When light passes through a single slit whose width w is on the order of the wavelength of the light, then we can observe a single slit diffraction pattern on a screen that is a distance L >> w away from the slit. The intensity is a function of angle. Huygens' principle tells us that each part of the slit can be thought of as an emitter of waves. All these waves interfere to produce the diffraction pattern. Where crest meets crest we have constructive interference and where crest meets trough we have destructive interference.
Very far from a point source the wave fronts are essentially plane waves. This is called the Fraunhofer regime, and the diffraction pattern is called Fraunhofer diffraction. The positions of all maxima (constructive interference) and minima (destructive interference) in the Fraunhofer diffraction pattern can be calculated fairly easily.
The Fraunhofer approximation, however, is only valid when the source, aperture, and detector are all very far apart or when lenses are used to convert spherical waves into plane waves. Being very far apart means that the distances between source, aperture, and detector must be much greater than the width of the aperture.
The Fresnel regime is the near-field regime. In this regime the wave fronts are curved, and their mathematical description is more involved.
The positions of all maxima and minima in the Fraunhofer diffraction pattern from a single slit can be found from the following simple arguments.
- Consider a slit of width w, as shown in the diagram on the right. A plane wave is incident from the bottom and all points oscillate in phase inside the slit.
- For light leaving the slit in a particular direction defined by the angle θ, we may have destructive interference between the ray at the right edge (ray 1) and the middle ray (ray 7). To arrive at a distant screen perpendicular to the direction of propagation of the rays, the rays coming from different points inside the slit have to travel different distances. They have a different optical path length. If ray 7 has to travel an extra distance of one-half wavelength (λ/2) compared to ray 1, then ray 1 and ray 7 destructively interfere. Crest meets trough.
- The optical path length (OPL) of a light ray traveling from point A to point B is defined as c times the time it takes the ray to travel from A to B. In free space the optical path length is just the distance d between the points. In a transparent medium with index of refraction n, it equals n times this distance, OPL = nd, because the light moves with speed c/n.
If the optical path length of two rays differs by λ/2, the two rays interfere destructively. For ray 1 and ray 7 to be half a wavelength out of phase we need
(w/2)sinθ = λ/2 or w sinθ = λ.

But from geometry, if these two rays interfere destructively, so do rays 2 and 8, 3 and 8, and 6 and 10, 5 and 11, and 6 and 12.
In effect, light from one half of the opening interferes destructively and cancels out light from the other half.
Destructive interference produces the dark fringes. Dark fringes in the diffraction pattern of a single slit are found at angles θ for which
w sinθ = mλ,
where m is an integer, m = 1, 2, 3, .. . For the first dark fringe we have w sinθ = λ.
When w is smaller than λ , the equation w sinθ = λ has no solution and no dark fringes are produced.
If the interference pattern is viewed on a screen a distance L from the slits, then the wavelength can be found from the spacing of the fringes. We have λ = w sinθ/m and sinθ = z/(L2 + z2)½), or
λ = zw/(m(L2 + z2)½),
where z is the distance from the center of the interference pattern to the mth dark line in the pattern.
If L >> z then (L2 + z2)½ ~ z/L and we can write
λ = zw/(mL).
Fresnel Diffraction And Fraunhofer Diffraction Pattern
Twitter rimworld. Please watch: Diffraction of Light - Exploring Wave Motion (YouTube)
Link: Diffraction Demo: Single Slit and Circular Aperture (Youtube)
Problem:
When a monochromatic light source shines through a 0.2 mm wide slit onto a screen 3.5 m away, the first dark band in the pattern appears 9.1 mm from the center of the bright band. What is the wavelength of the light?
Solution:
- Reasoning:
Dark fringes in the diffraction pattern of a single slit are found at angles θ for which w sinθ = mλ, where m is an integer, m = 1, 2, 3, .. . For the first dark fringe we have w sinθ = λ. Here we are asked to solve this equation for λ. - Details of the calculation:
z = 9.1 mm = 9.1*10-3 m.
L = 3.5 m.
w = 0.2 mm = 2*10-4 m.
L >> z, therefore sinθ ~ z/L and λ = zw/(mL).
λ = (9.1*10-3 m)(2*10-4 m)/(3.5 m).
λ = 5.2*10-7 m = 520 nm.
Problem:
Consider a single slit diffraction pattern for a slit width w. It is observed that for light of wavelength 400 nm the angle between the first minimum and the central maximum is 4*10-3 radians. What is the value of w?
Daz3d best iray render settings. Solution:
- Reasoning:
Dark fringes in the diffraction pattern of a single slit are found at angles θ for which w sinθ = mλ, where m is an integer, m = 1, 2, 3, .. . For the first dark fringe we have w sinθ = λ. Here we are asked to solve this equation for w. - Details of the calculation:
First minimum: w sinθ = λ, w = (400 nm)/sin(4*10-3 radians) = 1*10-4 m.

Diffraction is the bending of light when light encounters an obstacle of size which is in the range of wavelength of light. Hoping that you are clear with the basic idea of diffraction, lets move ahead. Based on the natural limits of diffraction. Expressions describing the vortex beams that are generated by the process of Fresnel diffraction of a Gaussian beam incident out of waist on fork-shaped gratings of arbitrary integer charge p, and vortex spots in the case of Fraunhofer diffraction by these gratings, are deduced. When calculating diffraction patterns, we further distinguish between near-field (Fresnel) and far-field (Fraunhofer) diffraction. In the far-field approximation, the source and observation screen are sufficiently far away from the diffracting aperture that we can treat the wavefronts as plane.
In classical physics, we can classify optical phenomena into one of two categories: ray optics and wave optics.
Light is a transverse electromagnetic wave. In many situations, the wavelengths of the light being studied are very small compared to the dimensions of the equipment used to study the light. Under these conditions we can make an approximation called geometrical optics or ray optics. Consider the wavelength scale of light waves. Wavelengths in the middle of the visible band are on the order of 500 nm. So a laser beam with a diameter of 1 mm has a diameter of 2000 wavelengths. Individual atoms in a solid are separated by distance on the order of 0.1 nm. So, as far as visible light is concerned, matter is quasi-continuous.
If the wavelengths of the light become comparable to the dimensions of the equipment, then we study optical phenomena using the classical theory of radiation, or wave optics. Wave optics contains all of ray optics, but the mathematical treatment is much more involved.
Diffraction and interference are phenomena observed with all waves. Diffraction can only be observed with waves traveling in two or three dimensions. (We already encountered interference when studying mechanical waves on a string and sound waves in physics 221.)
Diffraction is the tendency of a wave emitted from a finite source or passing through a finite aperture to spread out as it propagates. Diffraction results from the interference of an infinite number of waves emitted by a continuous distribution of source points in two or three dimensions. Huygens' principle lets us treat wave propagation by considering every point on a wave front to be a secondary source of spherical wavelets. These waveletspropagate outward with the characteristic speed of the wave. The wavelets emitted by all points on the wave front interfere with each other to produce the traveling wave. Huygens' principle also holds for electromagnetic waves. When studying the propagation of light, we can replace any wave front by a collection of sources distributed uniformly over the wave front, radiating in phase.
When light passes through a small opening, comparable in size to the wavelength λ of the light, in an otherwise opaque obstacle, the wave front on the other side of the opening resembles the wave front shown on the right.
The light spreads around the edges of the obstacle. This is the phenomenon of diffraction. Diffraction is a wave phenomenon and is also observed with water waves in a ripple tank.
Water waves in a ripple tank
| A single large slit | A single small slit |
Please watch: Apertures and Diffraction - Exploring Wave Motion (YouTube)
The single slit
When light passes through a single slit whose width w is on the order of the wavelength of the light, then we can observe a single slit diffraction pattern on a screen that is a distance L >> w away from the slit. The intensity is a function of angle. Huygens' principle tells us that each part of the slit can be thought of as an emitter of waves. All these waves interfere to produce the diffraction pattern. Where crest meets crest we have constructive interference and where crest meets trough we have destructive interference.
Very far from a point source the wave fronts are essentially plane waves. This is called the Fraunhofer regime, and the diffraction pattern is called Fraunhofer diffraction. The positions of all maxima (constructive interference) and minima (destructive interference) in the Fraunhofer diffraction pattern can be calculated fairly easily.
The Fraunhofer approximation, however, is only valid when the source, aperture, and detector are all very far apart or when lenses are used to convert spherical waves into plane waves. Being very far apart means that the distances between source, aperture, and detector must be much greater than the width of the aperture.
The Fresnel regime is the near-field regime. In this regime the wave fronts are curved, and their mathematical description is more involved.
The positions of all maxima and minima in the Fraunhofer diffraction pattern from a single slit can be found from the following simple arguments.
- Consider a slit of width w, as shown in the diagram on the right. A plane wave is incident from the bottom and all points oscillate in phase inside the slit.
- For light leaving the slit in a particular direction defined by the angle θ, we may have destructive interference between the ray at the right edge (ray 1) and the middle ray (ray 7). To arrive at a distant screen perpendicular to the direction of propagation of the rays, the rays coming from different points inside the slit have to travel different distances. They have a different optical path length. If ray 7 has to travel an extra distance of one-half wavelength (λ/2) compared to ray 1, then ray 1 and ray 7 destructively interfere. Crest meets trough.
- The optical path length (OPL) of a light ray traveling from point A to point B is defined as c times the time it takes the ray to travel from A to B. In free space the optical path length is just the distance d between the points. In a transparent medium with index of refraction n, it equals n times this distance, OPL = nd, because the light moves with speed c/n.
If the optical path length of two rays differs by λ/2, the two rays interfere destructively. For ray 1 and ray 7 to be half a wavelength out of phase we need
(w/2)sinθ = λ/2 or w sinθ = λ.
But from geometry, if these two rays interfere destructively, so do rays 2 and 8, 3 and 8, and 6 and 10, 5 and 11, and 6 and 12.
In effect, light from one half of the opening interferes destructively and cancels out light from the other half.
Destructive interference produces the dark fringes. Dark fringes in the diffraction pattern of a single slit are found at angles θ for which
w sinθ = mλ,
where m is an integer, m = 1, 2, 3, .. . For the first dark fringe we have w sinθ = λ.
When w is smaller than λ , the equation w sinθ = λ has no solution and no dark fringes are produced.
If the interference pattern is viewed on a screen a distance L from the slits, then the wavelength can be found from the spacing of the fringes. We have λ = w sinθ/m and sinθ = z/(L2 + z2)½), or
λ = zw/(m(L2 + z2)½),
where z is the distance from the center of the interference pattern to the mth dark line in the pattern.
If L >> z then (L2 + z2)½ ~ z/L and we can write
λ = zw/(mL).
Fresnel Diffraction And Fraunhofer Diffraction Pattern
Twitter rimworld. Please watch: Diffraction of Light - Exploring Wave Motion (YouTube)
Link: Diffraction Demo: Single Slit and Circular Aperture (Youtube)
Problem:
When a monochromatic light source shines through a 0.2 mm wide slit onto a screen 3.5 m away, the first dark band in the pattern appears 9.1 mm from the center of the bright band. What is the wavelength of the light?
Solution:
- Reasoning:
Dark fringes in the diffraction pattern of a single slit are found at angles θ for which w sinθ = mλ, where m is an integer, m = 1, 2, 3, .. . For the first dark fringe we have w sinθ = λ. Here we are asked to solve this equation for λ. - Details of the calculation:
z = 9.1 mm = 9.1*10-3 m.
L = 3.5 m.
w = 0.2 mm = 2*10-4 m.
L >> z, therefore sinθ ~ z/L and λ = zw/(mL).
λ = (9.1*10-3 m)(2*10-4 m)/(3.5 m).
λ = 5.2*10-7 m = 520 nm.
Problem:
Consider a single slit diffraction pattern for a slit width w. It is observed that for light of wavelength 400 nm the angle between the first minimum and the central maximum is 4*10-3 radians. What is the value of w?
Daz3d best iray render settings. Solution:
- Reasoning:
Dark fringes in the diffraction pattern of a single slit are found at angles θ for which w sinθ = mλ, where m is an integer, m = 1, 2, 3, .. . For the first dark fringe we have w sinθ = λ. Here we are asked to solve this equation for w. - Details of the calculation:
First minimum: w sinθ = λ, w = (400 nm)/sin(4*10-3 radians) = 1*10-4 m.
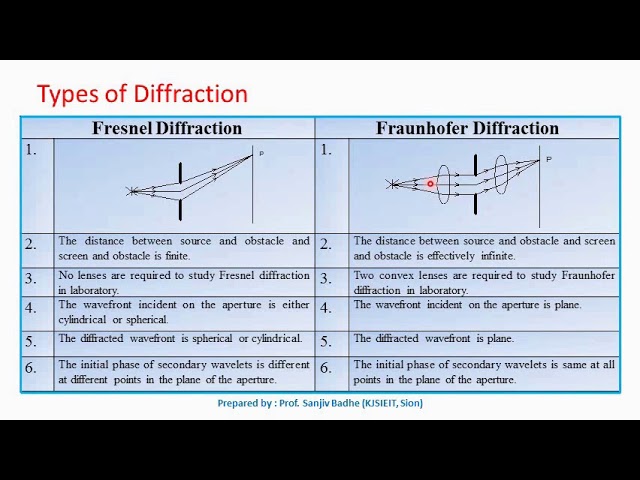
Distinguish, differentiate, compare and explain what is the difference between Fresnel and Fraunhofer diffraction. Comparison and Differences.
The spreading out of light wave into the geometrical shadow when it passes through a narrow opening is known as the diffraction. The difference between Fresnel and Fraunhofer diffraction is as follows:
Comparison and difference between Fresnel and Fraunhofer Diffraction
| S.No. | Fresnel Diffraction | Fraunhofer Diffraction |
| 1 | The source and the screen are at a finite distance from the diffracting aperture. | The source, and the screen is at an infinite distance from the diffracting aperture. |
| 2 | For obtaining Fresnel diffraction, zone plates are used. | The single-double plane diffraction grating is used. |
| 3 | The wave-fronts are divergent either spherical and cylindrical. | The wave-fronts are the plane which is realized by using the convex lens. |
| 4 | No mirror or lenses are used for observations. | Diffracted light is collected by a lens as in a telescope. |
According to Huygens, each point on a wavefront serves as the source of a spherical secondary wavelet with the same frequency as the primary wave. The amplitude at any point is the superposition of these wavelets.
Fraunhofer and Fresnel DiffractionConsider an opaque screen illuminated with a plane wave. The light spreads as a result of diffraction. If the observation screen is far enough away from the aperture, the diffraction pattern does not change in structure, but merely changes in size, as the distance is further increased. This situation is called Fraunhofer diffraction (shown in the image below).
Closer to the aperture the diffraction pattern does change with distance. This is called Fresnel diffraction. Calculation of Fresnel diffraction is based on an approximation, which eventually breaks down: closer to the aperture more advanced theories are required. The Fraunhofer diffraction pattern is obtained at a very large distance from the aperture, but using a lens, an image of it can be formed at a finite distance. In this case, the regions either closer or further from the lens give Fresnel diffraction.
Tags:
Fraunhofer Diffraction Theory
Difference between Fraunhofer Diffraction vs Fresnel
Fresnel vs Fraunhofer Diffraction
Fresnel Diffraction And Fraunhofer Diffraction Effect
Differences between Fraunhofer Diffraction vs Fresnel
